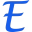In the diagram above, ABCD is a square with an area of 100 cm 2 and lines BD and AC are the diagonals of ABCD.
If line EF is parallel to line BC and the length of line CF = 3√ 2 cm, which of the following is equal to the shaded area?
A . 25 cm2
B . 39 cm2
C . 64 cm2
D . 78 cm2
E . 89 cm2
Answer: B
Explanation:
The area of a square is equal to S 2 where S is the length of one side of the square. A square with an area of 100 cm 2 has sides that are each equal to √100 = 10 cm. The diagonal of a square is equal to √2 times the length of a side of the square. Therefore, the lengths of diagonals AC and BD are 10√2 cm. Diagonals of a square bisect each other at right angles, so the lengths of segments OB and OC are each 5√ 2 cm. Since lines BC and EF are parallel and lines OC and OB are congruent, lines BE and CF are also congruent. The length of line OF is equal to the length of line OC plus the length of line CF: 5√2 + 3√ 2 = 8√ 2 cm. In the same way, OE = OB + BE = 5√2 + 3√2 = 8 √2 cm. The area of a triangle is equal to 1/2bh, where b is the base of the triangle and h is the height of the triangle. EOF is a right triangle, and its area is equal to 1/2 × 8√2 × 2√8 = 1/2 × 64 × 2 = 64 cm2. The size of the shaded area is equal to the area of EOF minus one-fourth of the area of ABCD: 64 C 1/4 × 100 = 64 C 25 = 39 cm 2 .
Latest ACT Math Dumps Valid Version with 280 Q&As
Latest And Valid Q&A | Instant Download | Once Fail, Full Refund