DELL EMC D-DS-FN-23 Dell Data Science Foundations 2023 Online Training
DELL EMC D-DS-FN-23 Online Training
The questions for D-DS-FN-23 were last updated at Jan 31,2025.
- Exam Code: D-DS-FN-23
- Exam Name: Dell Data Science Foundations 2023
- Certification Provider: DELL EMC
- Latest update: Jan 31,2025
Trend, seasonal, and cyclical are components of a time series.
What is another component?
- A . Irregular
- B . Linear
- C . Quadratic
- D . Exponential
Trend, seasonal, and cyclical are components of a time series.
What is another component?
- A . Irregular
- B . Linear
- C . Quadratic
- D . Exponential
Trend, seasonal, and cyclical are components of a time series.
What is another component?
- A . Irregular
- B . Linear
- C . Quadratic
- D . Exponential
Trend, seasonal, and cyclical are components of a time series.
What is another component?
- A . Irregular
- B . Linear
- C . Quadratic
- D . Exponential
Variable D is not significantly impacting the dependent variable.
After seeing your findings, the majority of your team agreed that variable B should be positively impacting the dependent variable.
What is a possible reason the coefficient for variable B was negative and not positive?
- A . Variable B is interacting with another variable due to correlated inputs
- B . Variable B needs a quadratic transformation due to its relationship to the dependent variable
- C . The information gain from variable B is already provided by another variable
- D . Variable B needs a logarithmic transformation due to its relationship to the dependent variable
Refer to the exhibit.
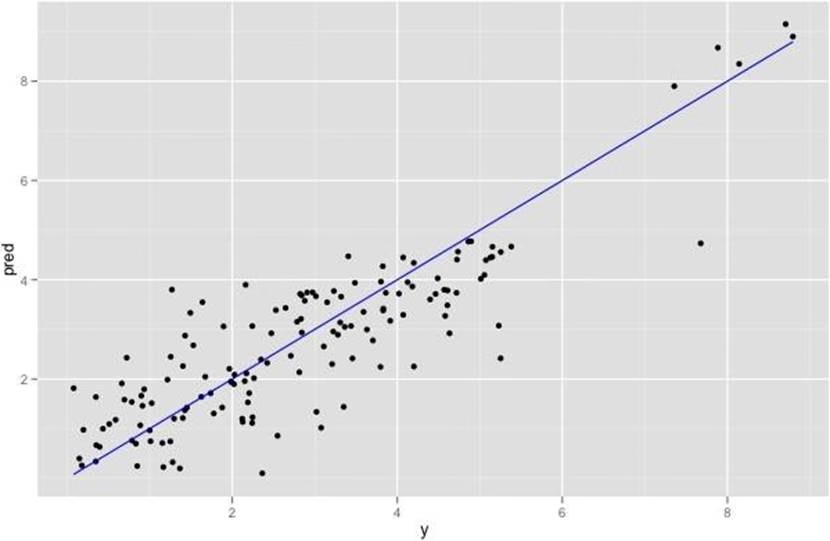
You have run a linear regression model against your data, and have plotted true outcome versus predicted outcome. The R-squared of your model is 0.75.
What is your assessment of the model?
- A . The R-squared may be biased upwards by the extreme-valued outcomes. Remove them and refit to get a better idea of the model’s quality over typical data.
- B . The R-squared is good. The model should perform well.
- C . The extreme-valued outliers may negatively affect the model’s performance. Remove them to see if the R-squared improves over typical data.
- D . The observations seem to come from two different populations, but this model fits them both equally well.
If distributed Item-based Collaborative Filtering is an algorithm supported by Mahout, what is the use case category of the algorithm?
- A . Classification
- B . Recommenders
- C . Frequent Itemset
- D . Clustering
Your risk analysis team has access to new customer financial data. You want to use this data to improve your prediction of credit default. Previously, the team was using only credit bureau scores, loan size, and customer income to assess risk of default.
What is the null hypothesis that should be used to evaluate the model?
- A . New model predicts as well as the toss of a coin weighted by the average default rate
- B . New model predicts better than the toss of a coin weighted by the average default rate
- C . Model using the new financial data predicts the outcome just as well as the previous model
- D . Model using the new financial data predicts the outcome better than the previous model
Which assumption makes the Naïve Bayesian classifier different from the general Bayesian model?
- A . Number of features cannot be greater than the number of records
- B . Features of a class are conditionally independent of one another
- C . All variables need to be numeric
- D . Fewer features can be used with the Naïve Bayes classifier
Refer to the exhibit.

You have plotted the distribution of savings account sizes for your bank.
How would you proceed, based on this distribution?
- A . The data is extremely skewed. Replot the data on a logarithmic scale to get a better sense of it.
- B . The data is extremely skewed, but looks bimodal; replot the data in the range 2, 500-10, 000 to be sure.
- C . The accounts of size greater than 2500 are rare, and probably outliers. Eliminate them from your future analysis.
- D . The data is extremely skewed. Split your analysis into two cohorts: accounts less than 2500, and accounts greater than 2500
Latest D-DS-FN-23 Dumps Valid Version with 300 Q&As
Latest And Valid Q&A | Instant Download | Once Fail, Full Refund

